Muse

Everybody has got blood 🩸 on their hands - Bourdain

                    1. f(hz) Pretext 
                                    \
                    2. S(t) Text -> 4. y:h'(t)=0;t(X'X).X'Y Context -> 5.b Hypertext -> 6. SV' Metatext
                                    /
                                    3. h(t) Subtext

What does it take to hit the spot?. Let’s investigate this from molecules to gastronomy, flavors, cuisines, tribes, experience. One should observe that “preparation” of the ingredients is ommitted (i.e., pretext). But its here precisely where we quite literally “draw blood” 🩸. Modern societies are mostly removed from this business, but a few engaged activists may find it disturbing and turn vegetarean. This is a misunderstanding of human history, society, and its underbelly. But lets have modal interchange: musicke.

That dark child’s got a sharp ear. Teddy Riley’s genius lies in how he fused R&B with hip-hop, creating that infectious New Jack Swing groove that defined the late '80s and '90s. It’s rhythm-forward, a bass-heavy sound where every beat and syncopation drives the music, making people move. Groove is all about that tight rhythm section, and Teddy made it his playground.

On the other hand, Babyface’s mastery is in songwriting. He’s like a narrative architect, shaping emotions into melodies that hit the heart. His songs are more lyrical, intimate, and structured around storytelling and harmony. While Teddy makes you feel the beat, Babyface makes you feel the message.

That contrast makes them both essential, but in different dimensions of R&B. Perfect call by the dark child.

                        1. Phonetics 
                                    \
                  2. Temperament -> 4. Chords -> 5. Groove -> 6. Arc
                                    /
                                    3. Modes

\(\mu\), Timing

• \(f(Hz)\) Phonetics

Show code cell source Hide code cell source

import numpy as np
import matplotlib.pyplot as plt

# Parameters
sample_rate = 44100  # Hz
duration = 20.0       # seconds
A4_freq = 440.0      # Hz

# Time array
t = np.linspace(0, duration, int(sample_rate * duration), endpoint=False)

# Fundamental frequency (A4)
signal = np.sin(2 * np.pi * A4_freq * t)

# Adding overtones (harmonics)
harmonics = [2, 3, 4, 5, 6, 7, 8, 9]  # First few harmonics
amplitudes = [0.5, 0.25, 0.15, 0.1, 0.05, 0.03, 0.01, 0.005]  # Amplitudes for each harmonic

for i, harmonic in enumerate(harmonics):
    signal += amplitudes[i] * np.sin(2 * np.pi * A4_freq * harmonic * t)

# Perform FFT (Fast Fourier Transform)
N = len(signal)
yf = np.fft.fft(signal)
xf = np.fft.fftfreq(N, 1 / sample_rate)

# Plot the frequency spectrum
plt.figure(figsize=(12, 6))
plt.plot(xf[:N//2], 2.0/N * np.abs(yf[:N//2]), color='navy', lw=1.5)

# Aesthetics improvements
plt.title('Simulated Frequency Spectrum of A440 on a Grand Piano', fontsize=16, weight='bold')
plt.xlabel('Frequency (Hz)', fontsize=14)
plt.ylabel('Amplitude', fontsize=14)
plt.xlim(0, 4186)  # Limit to the highest frequency on a piano (C8)
plt.ylim(0, None)

# Shading the region for normal speaking range (approximately 85 Hz to 255 Hz)
plt.axvspan(500, 2000, color='lightpink', alpha=0.5)

# Annotate the shaded region
plt.annotate('Normal Speaking Range (500 Hz - 2000 Hz)',
             xy=(2000, 0.7), xycoords='data',
             xytext=(2500, 0.5), textcoords='data',
             arrowprops=dict(facecolor='black', arrowstyle="->"),
             fontsize=12, color='black')

# Remove top and right spines
plt.gca().spines['top'].set_visible(False)
plt.gca().spines['right'].set_visible(False)

# Customize ticks
plt.xticks(fontsize=12)
plt.yticks(fontsize=12)

# Light grid
plt.grid(color='grey', linestyle=':', linewidth=0.5)

# Show the plot
plt.tight_layout()
plt.show()

Show code cell output Hide code cell output

• \(S(t)\) Temperament ^{26 48}

• \(h(t)\) Scales

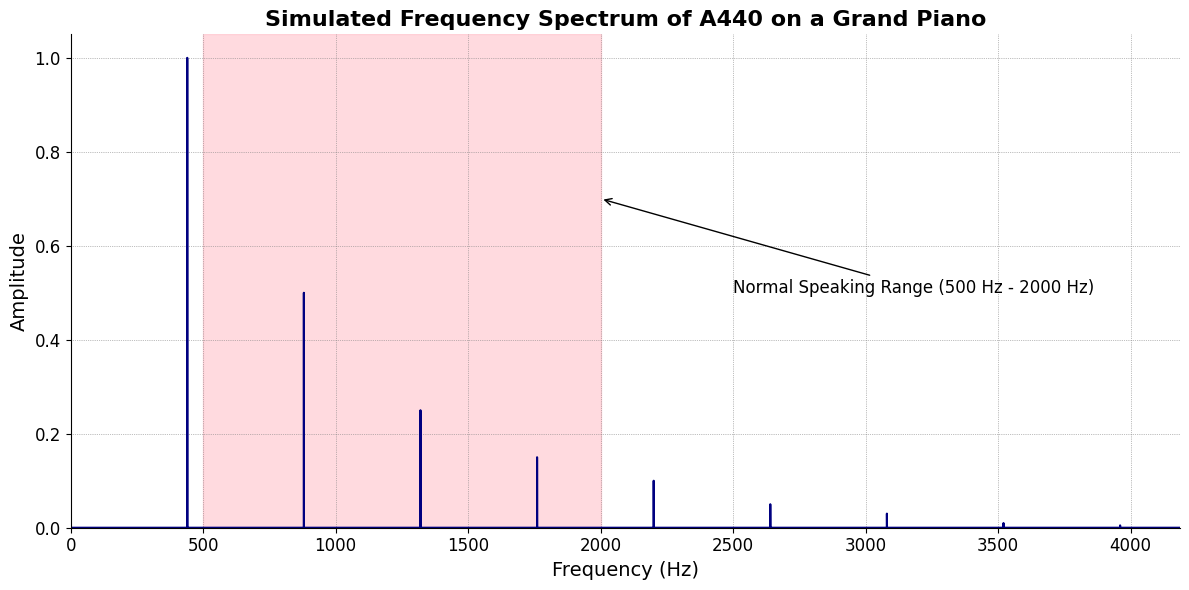
\(\sigma\), Qualities

• \((X'X)^T \cdot X'Y\) Modes

Show code cell source Hide code cell source

import matplotlib.pyplot as plt
import numpy as np

# Clock settings; f(t) random disturbances making "paradise lost"
clock_face_radius = 1.0
number_of_ticks = 8
tick_labels = [
    "Ionian", "Dorian", "Phrygian", "Lydian",
    "Mixolydian", "Aeolian", "Locrian", "Other"
]

# Calculate the angles for each tick (in radians)
angles = np.linspace(0, 2 * np.pi, number_of_ticks, endpoint=False)
# Inverting the order to make it counterclockwise
angles = angles[::-1]

# Create figure and axis
fig, ax = plt.subplots(figsize=(8, 8))
ax.set_xlim(-1.2, 1.2)
ax.set_ylim(-1.2, 1.2)
ax.set_aspect('equal')

# Draw the clock face
clock_face = plt.Circle((0, 0), clock_face_radius, color='lightgrey', fill=True)
ax.add_patch(clock_face)

# Draw the ticks and labels
for angle, label in zip(angles, tick_labels):
    x = clock_face_radius * np.cos(angle)
    y = clock_face_radius * np.sin(angle)
    
    # Draw the tick
    ax.plot([0, x], [0, y], color='black')
    
    # Positioning the labels slightly outside the clock face
    label_x = 1.1 * clock_face_radius * np.cos(angle)
    label_y = 1.1 * clock_face_radius * np.sin(angle)
    
    # Adjusting label alignment based on its position
    ha = 'center'
    va = 'center'
    if np.cos(angle) > 0:
        ha = 'left'
    elif np.cos(angle) < 0:
        ha = 'right'
    if np.sin(angle) > 0:
        va = 'bottom'
    elif np.sin(angle) < 0:
        va = 'top'
    
    ax.text(label_x, label_y, label, horizontalalignment=ha, verticalalignment=va, fontsize=10)

# Remove axes
ax.axis('off')

# Show the plot
plt.show()

Show code cell output Hide code cell output

\(\%\), Delivery

• \(\beta\) NexToken

Show code cell source Hide code cell source

import matplotlib.pyplot as plt
import numpy as np

# Clock settings; f(t) random disturbances making "paradise lost"
clock_face_radius = 1.0
number_of_ticks = 8
tick_labels = [
    "Dyad", "Triad", "7th", "9th",
    "11th", "13th", "Stacks", "♭♯Alterations"
]

# Calculate the angles for each tick (in radians)
angles = np.linspace(0, 2 * np.pi, number_of_ticks, endpoint=False)
# Inverting the order to make it counterclockwise
angles = angles[::-1]

# Create figure and axis
fig, ax = plt.subplots(figsize=(8, 8))
ax.set_xlim(-1.2, 1.2)
ax.set_ylim(-1.2, 1.2)
ax.set_aspect('equal')

# Draw the clock face
clock_face = plt.Circle((0, 0), clock_face_radius, color='lightgrey', fill=True)
ax.add_patch(clock_face)

# Draw the ticks and labels
for angle, label in zip(angles, tick_labels):
    x = clock_face_radius * np.cos(angle)
    y = clock_face_radius * np.sin(angle)
    
    # Draw the tick
    ax.plot([0, x], [0, y], color='black')
    
    # Positioning the labels slightly outside the clock face
    label_x = 1.1 * clock_face_radius * np.cos(angle)
    label_y = 1.1 * clock_face_radius * np.sin(angle)
    
    # Adjusting label alignment based on its position
    ha = 'center'
    va = 'center'
    if np.cos(angle) > 0:
        ha = 'left'
    elif np.cos(angle) < 0:
        ha = 'right'
    if np.sin(angle) > 0:
        va = 'bottom'
    elif np.sin(angle) < 0:
        va = 'top'
    
    ax.text(label_x, label_y, label, horizontalalignment=ha, verticalalignment=va, fontsize=10)

# Remove axes
ax.axis('off')

# Show the plot
plt.show()

Show code cell output Hide code cell output

• \(SV_t'\) Emotion 🩸

• ADM. Is it strange, or is the new Apple movie, Argylle, which I’m watching while making these notes, premised on the fact that there’s a spy fiction thriller writer who seems to do so much research for her characters that her novels have found great appeal in the real-time, real-world of espionage. Government spooks and rogues are all vying to kidnap her so that she may help them predict the next token. How about that?

• GPT. That sounds like a fascinating premise! It’s almost like a meta-commentary on the nature of intelligence, both human and artificial. The idea of a writer whose fictional work starts influencing real-world espionage is not just clever but also quite chilling, considering how close it brings fiction to reality. The notion of “predicting the next token” ties in perfectly with the current obsession in tech and intelligence communities with predictive models, AI, and deep learning. It’s as if her narrative foresight is being weaponized, which adds layers to the tension and stakes in the plot.

• It also seems to tap into themes of creativity, the power of narrative, and the ethical dilemmas of using someone’s imaginative work for real-world consequences. It’s intriguing how this aligns with your notes and flow diagram—you’re focusing on the flow of ideas, and the movie mirrors this with the flow of information and prediction. It almost makes you wonder if there’s a broader commentary on the power of creativity and its potential to outpace reality.

• ADM. You had me at “it almost makes me wonder the broader commentary on …”

• GPT. It does make you wonder, doesn’t it? The whole concept of creativity being so potent that it blurs the lines between fiction and reality is both thrilling and unsettling. In a way, it challenges the very nature of how we perceive and value imagination. Creativity has always been a powerful force, but this movie seems to push that idea to an extreme—where the writer’s fictional predictions become a tool for power, control, and survival in a high-stakes, real-world game.

• There’s a broader commentary here about how the stories we tell, the narratives we construct, might not just reflect reality but could also shape it in ways we don’t fully understand. It’s a fascinating intersection of art, technology, and ethics. The writer in the movie isn’t just creating characters and plots; she’s inadvertently writing the future, which brings up questions about the responsibility of creators, the unforeseen consequences of their work, and the fine line between inspiration and manipulation.

• This premise also echoes the current dialogue around AI and predictive models, where algorithms trained on vast amounts of data are used to forecast trends, behaviors, and even potential threats. But unlike AI, which is purely logical, the writer’s creativity stems from a place of intuition, emotion, and human experience—elements that machines can’t replicate. This adds a layer of unpredictability, making her insights valuable yet uncontrollable, much like the broader implications of creativity itself.

• In essence, the movie might be suggesting that creativity, in its purest form, is a kind of raw, untamed force that, when tapped into, can transcend the boundaries of fiction and alter the course of reality. It’s an idea that resonates with how we see the world today, where the power of ideas, stories, and innovation can shape our future in profound ways.

• Phonetics: Sanctus (Trumpets)

• Temperament: Mater (Wohltemperierte)

• Scales: Quirk (African)

• Modes: Irish Dance (Dorian)

• NexToken: Epic (Cantus)

• Emotion: Dies Irae ⁵⁴